To run EXPO2014 for space group determination you can follow two different approach
- Creating an input file (*.exp). You can edit a new input file or modify an exiting input file by a text editor and save it with extension .exp.
A typical .exp input file for space group determination is here reported in case of the crystal structure determination of cimetidine from the indexed pattern.
%structure cime %job cimetidine (C10H16N6S) -- Synchrotron data %data pattern cime.dat wavelength 1.52904 synchrotron cell 10.69808 18.81786 6.82267 90.00000 111.28911 90.00000 findspace content (C10H16N6S)4 %continue
The directive findspace enables the space group determination procedure. It is a directive of the command %data. The unit cell is assumed to be determined by the indexing step, the directive synchrotron is necessary only in case of X-ray synchrotron diffraction data. The cell content (directive content) is mandatory for the space group determination. To know the meaning of the other lines of the input file, see the chapter command and their use of this manual. For more information about how you can create an .exp input file visit the Preparing input file section.
Once a time the input file has been created, load the .exp input file from menu File > Load & Go.
- By graphical interface. Load the powder diffraction data file selecting Import Diffraction Pattern in the menu File, then select Pattern > Space Group > Space Group Determination. Fill the frame ‘Cell Parameters’ and ‘Cell Content’.
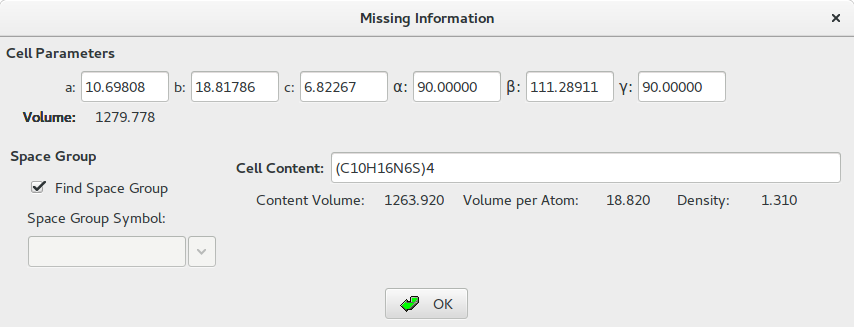 Press the OK button to launch the space group determination step.
Press the OK button to launch the space group determination step.
The different steps of the algorithm are here schematized.
1) The full pattern decomposition is performed, after that the integrated intensities have been extracted from the experimental pattern according to the Le Bail algorithm, in the space group having the largest Laue symmetry and no extinction conditions (e.g., P 1 2/m 1 in the monoclinic system, P 2/m 2/m 2/m for the orthorhombic, P 4/m m m for the tetragonal, P 6/m m m for the trigonal-hexagonal, P m -3 m for the cubic systems).
2) The extracted intensities are then normalized, according to the classical Wilson method, by using the space group mentioned at the point 1).
3) The statistic of the suitably weighted integrated intensities is used to define the probability of the extinction symbol.
4) For each crystal system the probability corresponding to the different possible extinction symbols is calculated.
Use the button ![]() on the toolbar to carry out all the next steps. At the end of the procedure the following window will be displayed containing the list of the most plausible space groups and the corresponding extinction symbol and probability (FoM).
on the toolbar to carry out all the next steps. At the end of the procedure the following window will be displayed containing the list of the most plausible space groups and the corresponding extinction symbol and probability (FoM).

In general, more space groups are associated to the same extinction symbol (e.g., P 2/n and P n in case of the extinction symbol P 1 n 1) but occasionally just one space group can correspond to an extinction symbol (e.g., P 21/n in case of the extinction symbol P 1 21/n 1).
Additional information reported in the window above can be useful in case of space group ambiguities: the number of systematically absent reflections not in overlap (Nabs), the expected number of atoms in the asymmetric unit (Nasym), the frequency of the selected space group in the Cambridge Structural Database (CSD) in terms of the number of occurrences, the percentage of occurrences in the CSD (% of CSD) and the space group frequency ranking (Rank). In the last column is reported ‘yes’ if the space group is a chiral (Sohnke) space group; this information is useful because enantiomerically pure compound can crystallize only in a chiral space group.
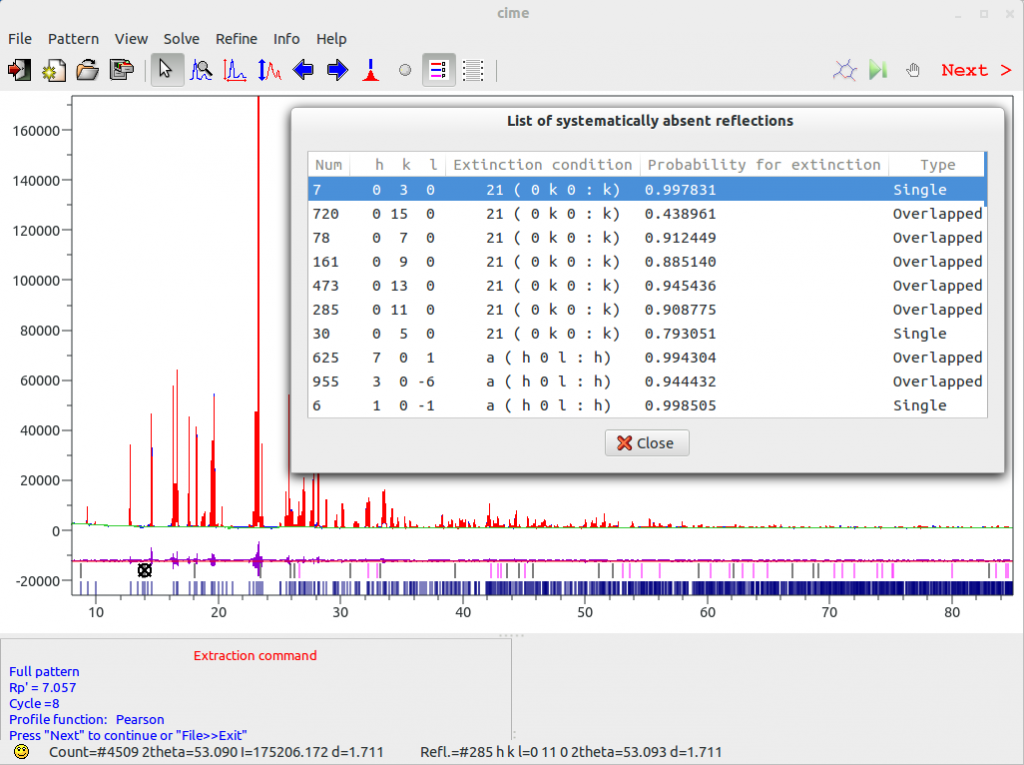
Press the button ![]() to access to a list of reflections that, according to the selected extinction symbol, should be systematically absent. For each reflection the following specifications are given: the order number of the reflection, the Miller indices, the symbol of the symmetry operator responsible for the extinction and the corresponding extinction condition, the probability of the extinction, the type of reflection (single or in overlap).
to access to a list of reflections that, according to the selected extinction symbol, should be systematically absent. For each reflection the following specifications are given: the order number of the reflection, the Miller indices, the symbol of the symmetry operator responsible for the extinction and the corresponding extinction condition, the probability of the extinction, the type of reflection (single or in overlap).
By default the reflections are ordered according to the extinction condition but you can change the order selecting a different ordering criterion by clicking on the title column. The selected reflection on the list will be marked on the pattern.
The reflections that are expected to be absent according to the selected extinction symbol are marked by vertical bars located below the observed pattern, just above the set of bars showing the positions of reflections. They have different colors depending on whether the reflection is single (or belongs to a whole extinct cluster) or in overlapping. By default, a black vertical bar marks single systematically absent reflections, while the rest of the absent reflections (those in overlap) are marked with a purple bar. This graphical support helps the user to discard unreliable extinction symbols even if estimated with large probability.
Sometimes this procedure doesn’t guarantee that the space group corresponding to the highest FoM is the correct one, at the same time space groups having identical systematic absences (corresponding to the same extinction symbol) cannot be distinguished. In case of ambiguities more space groups should be for the structure solution step. A good choice is to start from the space group with the highest frequency.
Press the button ![]() to accept the selected space group and continue with the structure solution process. You can also save the table of results in an .expo project file selecting File > Save Project As. To reload the .expo file click on File > Old Project.
to accept the selected space group and continue with the structure solution process. You can also save the table of results in an .expo project file selecting File > Save Project As. To reload the .expo file click on File > Old Project.
The output file contains details about the space group determination procedure. Use Info > View output file to access to the output file or locate the file structure_name.out in your working directory and open it with a text editor. At the end of the procedure a new input file, called structure_name1.exp (e.g. cime1.exp) will be created. This file contains the selected space group and can be loaded to skip the space group determination and to perform the structure solution process. The table of space groups is accessible by selecting Pattern > Space Group > Space Group List.
Altomare, A., Caliandro, R., Camalli, M., Cuocci, C., da Silva, I., Giacovazzo, C., Moliterni, A. G. G. & Spagna, R. (2004). Space-group determination from powder diffraction data: a probabilistic approach. J. Appl. Cryst. 37, 957-966.
Altomare, A., Camalli, M., Cuocci, C., da Silva, I. Giacovazzo, C., Moliterni, A. G. G & Rizzi, R. (2005). Space group determination: improvements in EXPO2004. J. Appl. Cryst. 38, 760-767.
Altomare, A., Camalli, M., Cuocci, C., Giacovazzo, C., Moliterni, A. G. G. and Rizzi, R. (2007). Advances in space-group determination from powder diffraction data. J. Appl. Cryst. 40, 743-748.